
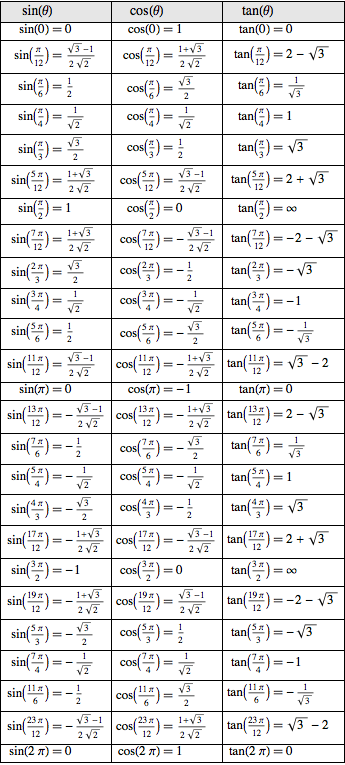
Mathematica also automatically simplifies the composition of the direct and any of the inverse trigonometric functions into algebraic functions of the argument. Mathematica automatically simplifies the composition of the direct and the inverse tangent functions into its argument. Mathematica knows symmetry and periodicity of the tangent function. The remaining digits are suppressed, but can be displayed using the function InputForm. In this case, only six digits after the decimal point are shown in the results. Mathematica automatically evaluates mathematical functions with machine precision, if the arguments of the function are machine‐number elements. Here is a 50‐digit approximation of the tangent function at the complex argument. The next input calculates 10000 digits for and analyzes the frequency of the digit in the resulting decimal number. Within a second, it is possible to calculate thousands of digits for the tangent function. The next inputs calculate 100‐digit approximations at and. Here are three examples: CForm, TeXForm, and FortranForm.Īutomatic evaluations and transformationsĮvaluation for exact, machine-number, and high-precision argumentsįor the exact argument, Mathematica returns an exact result.įor a machine‐number argument (a numerical argument with a decimal point and not too many digits), a machine number is also returned. Mathematica also knows the most popular forms of notations for the tangent function that are used in other programming languages. This shows the tangent function in TraditionalForm. This shows the tangent function in StandardForm. These involve numeric and symbolic calculations and plots.įollowing Mathematica's general naming convention, function names in StandardForm are just the capitalized versions of their traditional mathematics names. Examples of evaluating Mathematica functions applied to various numeric and exact expressions that involve the tangent function or return it are shown.
The following shows how the tangent function is realized in Mathematica. I hope this example is useful in terms of this discussion.Introduction to the Tangent Function in Mathematica Not as "cut and dried" as I had expected! a mathematical function seems to be context dependent. I've Googled various sources on the web and the distinction between a mathematical operator vs. The debate expanded to include a debate on exponentiation (operation vs. Nearly $400$ ensuing comments were posted in a debate about what constitutes an operator versus a function. This was immediately challenged by someone who stated that the factorial symbol $\,(!)\,$ represents a function rather than an operation and therefore the proposed answer violates the original requirement that specifies the use of operations. $$1 \left\langle \,\Rule\,\right\rangle\,$ represents the space for an operator Perhaps this can serve as an example for this discussion, if I'm interpreting the original question correctly, that is: Yes, this is how we start off learning about functions, but we quickly move on to functions of more than one input (those whose graphs describe surfaces, for example). are applied.Ī footnote: at least one of the answers above implied that a function, unlike an operator, has only one input. "How are the words 'operator' and 'function' (and, if you like, 'mapping', 'transformation') used when we are talking about mathematics?" Īnother way of approaching it - really, the same idea as the above but starting from the other end, so to speak - is to ask about the underlying mathematical reality (in this case, values which are being transformed to yield new values), to which the words "operator", "function" etc. To answer this question, may I suggest that everyone think like this: instead of asking " IS an operator a function?" or "What IS an operator?" etc.


 0 kommentar(er)
0 kommentar(er)
